LC Oscillations

An oscillating $\mathrm{LC}$circuit consists of a $75 \mathrm{mH}$ inductor and a $1.2 \mu \mathrm{F}$ capacitor. If the maximum charge to the capacitor is $2.7 \mu \mathrm{C}$. The maximum current in the circuit will be $\mathrm{\_\_\_\_\_\_\_} \mathrm{mA}$.

In an LC oscillating circuit with $L=75 \mathrm{mH}$ and $C=30 \mathrm{\mu F}$, the maximum charge of capacitor is $2.7\times {10}^{-4} \mathrm{C}$. Maximum current through the circuit will be

In the circuit shown in figure $S_1$ is open and, $S_2$ and $S_3$ are closed. The circuit is in steady state. At time $t=0$, switch $S_1$, is closed and $S_2$ and $S_3$ are opened simultaneously. $V=100 \mathrm{V}$, $R=10 \mathrm{\Omega }, C=100 \mathrm{\mu F}, L=0.03 \mathrm{H}$

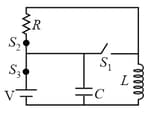
Find the maximum charge that will appear on the capacitor at any time

A capacitor of capacitance $0.6 \mu \mathrm{F}$ is charged by a $6 \mathrm{V}$ battery. Charged capacitor is now connected to an inductor of inductance $2 \mathrm{mH}$. Then find the current in the circuit when one third energy stored in capacitor converts into the energy stored in inductor.

In L.C oscillation, maximum current in the inductor can be $I$. If at any instant, electric energy and magnetic energy associated with circuit is equal, then current in the inductor at that instant is

In an L-C oscillation maximum charge on capacitor can be $Q_0$. If at any instant electrical energy is $\frac{3}{4}$th of the magnetic energy then the charge on capacitor at that instant is

When current in coil changes from $5 \mathrm{A}$ to $2 \mathrm{A}$ in $0.1 \mathrm{s}$, an average of $50 \mathrm{V}$ is produced. The self-inductance in $\mathrm{Henry}\left(\mathrm{H}\right)$ of the coil is $\frac{n}{3}$. Write the value of $n$.

A $60\mu \mathrm{F}$ capacitor is charged to $100 \mathrm{volts}$. This charged capacitor is connected across a $1.5\mathrm{mH}$ coil, so that $\mathrm{LC}$ oscillations occur. The maximum current in the coil is:-

In an $LC$ circuit shown in figure, $C=2 \mathrm{F}$ and $L=2 \mathrm{H}$. At time $t=0$, charge on the capacitor is $3$ coulomb and it is decreasing with rate of $\sqrt{4} \mathrm{C} {\mathrm{s}}^{-1}$. Then choose the correct statement.

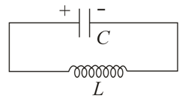
The natural frequency of the circuit is (in $\mathrm{rad} {\mathrm{s}}^{-1}$),

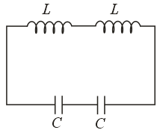
A charged $30 \mathrm{\mu }\mathrm{F}$ capacitor is connected to a $27 \mathrm{m}\mathrm{H}$ inductor. The angular frequency of free oscillations of the circuit is

A $1.5\mu \mathrm{F}$ capacitor is charged to $60\mathrm{V}$ and a$15\mathrm{mH}$ coil is connected across it. Assuming that the circuit contains no resistance, what will be the maximum current in the coil?

A capacitor of capacitance $100 \mathrm{\mu F}$ is charged to a potential of $12 \mathrm{V}$ and connected to a $6.4 \mathrm{mH}$ inductor to produce oscillations. The maximum current in the circuit would be :

A capacitor of capacitance $100 \mathrm{\mu F}$ is charged to a potential of $12 \mathrm{V}$ and is connected to an inductor of inductance $6.4 \mathrm{mH}$. What is the maximum current through the inductor at resonance?

A $1.5 \mathrm{\mu }\mathrm{F}$ capacitor is charged to $60 \mathrm{V}$. The charging battery is then disconnected and a $15 \mathrm{m}\mathrm{H}$ coil is connected in series with the capacitor so that $\mathrm{LC}$ oscillations occur. Assuming that the circuit contains no resistance, the maximum current in this coil shall be close to

An $LC$ circuit contains a $20\mathrm{ }\mathrm{m}\mathrm{H}$ inductor and a $50 \mathrm{\mu }\mathrm{F}$ capacitor with an initial charge of $10\mathrm{ }\mathrm{mC}$. The resistance of the circuit is negligible. Let the instant at which the circuit which is closed be $t=0$. At what time the energy stored is completely magnetic?

A circuit has a self inductance of $1$ henry and carries a current of $2 \mathrm{A}$. To prevent sparking when the circuit is broken, a capacitor which can withstand $400 \mathrm{volts}$ is used. The least capacitance of the capacitor connected across the switch must be equal to

The natural frequency of an LC circuit is 125 kHz. When the capacitor is totally filled with a dielectric material, the natural frequency decreases by 25 kHz. Dielectric constant of the material is nearly

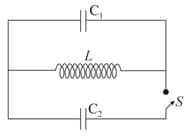
At a moment $\left(t=0\right),$ when the charge on capacitor $C_1$ is zero, the switch is closed. If $I_0$ be the current through the inductor at $t=0$, for $\mathrm{ }t>0$ (initially, $C_2\mathrm{ }$is uncharged.)

The tuning circuit of a radio receiver has a resistance of $50 \mathrm{\Omega }$, an inductor of $10\mathrm{ }\mathrm{m}\mathrm{H}$ and a variable capacitor. $1\mathrm{ }\mathrm{M}\mathrm{H}\mathrm{z}$ radio wave produces a potential difference of $0.1\mathrm{ }\mathrm{m}\mathrm{V}$. The values of the capacitor to produce resonance is (Take ${\mathrm{\pi }}^2=10$)